Written on: January 1, 2019 by W. Stephen Tait
Hello, everyone and welcome to the New Year. There are 14 possible types of corrosion in the most widely used spray package components as illustrated in Figure 1 and Figure 2. These two Figures are from the Pair O Docs primer on spray package corrosion science and corrosion testing (copies available on request).
Figure 1: Possible forms of traditional aerosol container corrosion
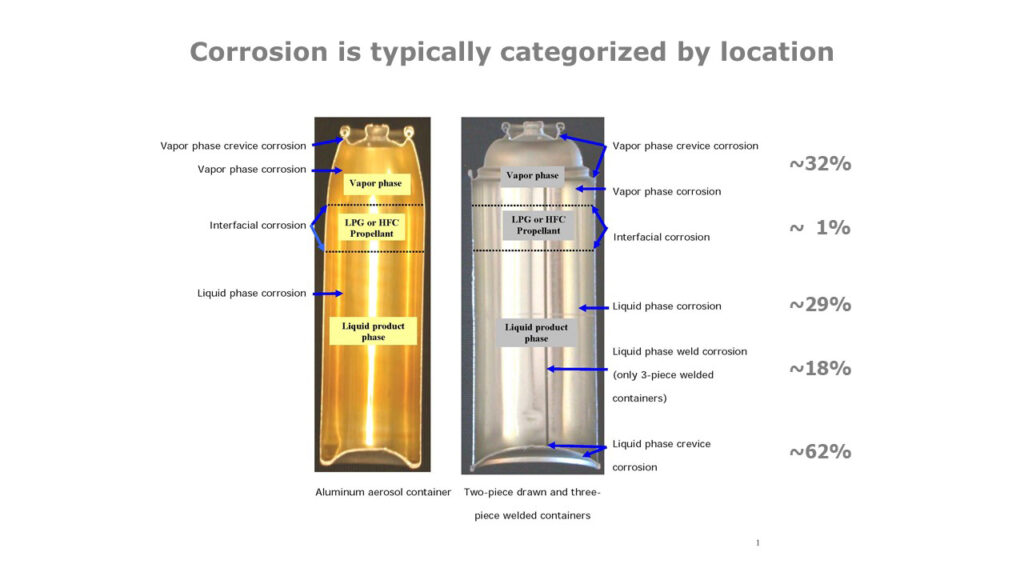
Figure 2: Possible forms of corrosion for laminated aluminum foil and aerosol valve 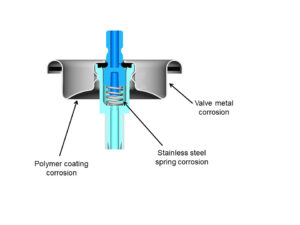 corrosion
corrosion

Corrosion testing is the most reliable way to determine:
It takes time to conduct corrosion tests properly and I’m often asked if there are mathematical equations that can be used to predict if corrosion will occur and what is the container service lifetime without having to conduct corrosion tests. It is theoretically possible to mathematically model spray package corrosion from first-principles. However, the number of factors that influence corrosion and the types of spray packaging available make the models (or equations) complex.
Corrosion of materials occurs when the Gibbs free energy for a corrosion reaction is negative. The theoretical equation for this principle is:
ΔG = -n F (corrosion potential)
• ΔG is the symbol for the Gibbs free energy
• n is the number of electrons in the metal corrosion reaction
• F is a conversion factor known as the Faraday’s constant
The corrosion reactions for the two types of spray package base
metals are:
• Fe0 Fe+2 2e- (tinplated steel, tin-free-steel and spring
corrosion)
• Al0 Al+3 3e- (aluminum and aluminum foil corrosion)
The magnitude of ΔG is determined by the:
• Formula or contaminant water pH
• Types of package metal
• Formula/package surface tension
• Chemical activity for each electrochemically active (ECA) ion
and molecule in a formula
• Surface condition of the spray package metal or metal foil
(e.g., un-coated, coated or laminated)
Thus, an empirical version of the theoretical Gibbs equation that accounts for these five factors is:
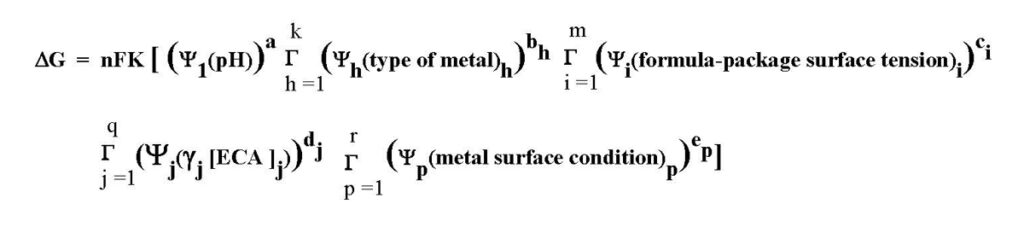
The “n” and “F” symbols are the same as those in the theoretical Gibbs free energy equation and the “K” is a conversion constant. The Ψ symbol represents the probability for individual corrosion factors. The G symbol indicates a combination of several sub factors multiplied together.
Lower-case superscript letters are exponents for individual terms in the equation. For example, in the term Y1(pH)a the “a” is an exponent that could be from zero or greater than zero. The exponent magnitude determines how much a factor influences whether or not corrosion will occur (pH in this example).
Subscript lower-case letters in each term of the equation are numbers ranging from one to the actual number of subfactors that determine if corrosion will or will not occur. For example, in the term:
k
Γ(Ψh (type of metal) h) bh
h =1
The “h=1” and “k” below and above the G, respectively, indicate there are from one to k subfactors that are multiplied together to determine whether or not corrosion will occur
An empirical Gibbs equation could be used to determine if corrosion will occur and how much influence each factor/sub-factor has in causing corrosion. However, all the exponents and parameters are needed for the empirical equation to be accurate. Unfortunately, most of the exponents and probabilities are either unknown at this time or not available in the public domain. In addition, the exponents and probabilities are specific for each different formula chemical composition.
Consequently, it is actually easier to measure the corrosion potential. However, it is essential that corrosion potentials are measured at steady state, because measuring non-steady state potentials will lead to erroneous conclusions about product corrosivity. Corrosion potentials should also be measured with a high impedance electrometer and not a voltmeter.
Measured corrosion potentials are composites and thus do not provide information about how many specific factors are contributing to or causing corrosion. Please remember that the corrosion potential only indicates if corrosion will occur and does not provide any information on how fast corrosion will occur.
Hopefully the empirical Gibbs equation provides an appreciation of the complexity of corrosion and why reviewing formula ingredients is not always a reliable way to determine if a formula will or will not cause spray package corrosion. Hopefully, the complexity of the empirical Gibbs equation also demonstrates why measurement is preferred over a review of formula ingredients.
The February edition of Corrosion Corner will discuss an empirical equation for predicting “how fast” corrosion will occur using first principles. Thanks for reading and I’ll see you in February. SPRAY